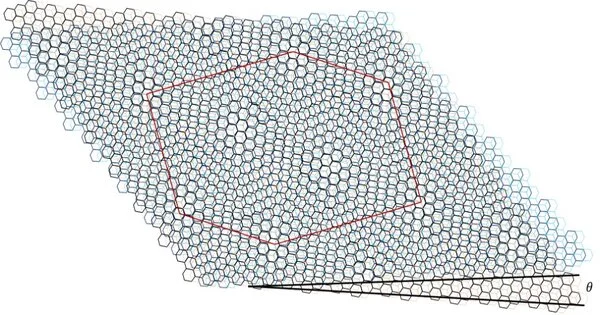
The Berry curve and Chern number are critical topological characteristics of a quantum mechanical beginning describing the electron wave capability of materials. These two components assume a vital part in deciding the properties of explicit materials.
While many examinations have attempted to decide what the Berry arch and Chern number mean for the properties of materials, distinguishing them in an exploratory setting can be undeniably challenging. Bent twofold bilayer graphene, a material that comprises of two stacked bilayer graphene precious stones, is an especially encouraging stage to control the Berry shape and valley Chern quantities of topological level groups and subsequently to concentrate on their belongings.
Specialists at Tata Institute of Fundamental Research, the Indian Institute of Technology, and Jawaharlal Nehru Center for Advanced Scientific Research have been analyzing the tunable properties of turned twofold bilayer graphene for over three years. In their latest review, highlighted in Nature Physics, they had the option to straightforwardly identify a topological progress in a moiré superlattice by controlling the sign change in the Berry ebb and flow dipole.
“When an external magnetic field is applied perpendicular to the sample’s plane, the Hall voltage usually develops perpendicular to the flow of current. Interestingly, due to topological bands, one can have a Hall voltage in the absence of a magnetic field even in non-magnetic materials, as demonstrated by Sodemann and Fu’s pioneering theoretical work, and we measured this voltage.”
Subhajit Sinha
This paper expands on Prof. Mandar Deshmukh’s past works zeroing in on bent twofold bilayer graphene. In one of their past examinations, for example, the scientists acquainted systems with identify the Berry ebb and flow, which they then applied in their new trials.
“Before we began dealing with this venture, Prof. Amit Agarwal’s gathering was hypothetically investigating different Hall commitments because of quantum mechanical impacts,” Subhajit Sinha, one of the analysts who did the review, told Phys.org. “On Christmas night before 2020, he kept in touch with us in regards to estimating the non-straight Hall impact in our examples. One of our wound twofold bilayer graphene tests was cold in a cryostat, so we chose to gather estimations on it and check whether we got anything. Maybe a few fates were lined up, on the grounds that we did to be sure quantify some sign!”
After they approved their underlying perceptions and estimations by playing out a few crosschecks, the group had the option to decide with a serious level of sureness that they had as a matter of fact estimated the non-direct quantum Hall impact in their turned twofold bilayer graphene test. They then, at that point, performed different examinations as a team with Prof. Amit’s exploration gathering to exhibit that they had straightforwardly noticed a topological progress.
In their new tests, Prof. Mandar’s gathering at TIFR explicitly estimated the nonlinear Hall voltage in their bent twofold bilayer graphene test. This is a nonlinear voltage that can be driven by an opposite in-plane electrical flow in the Hall-bar estimation.
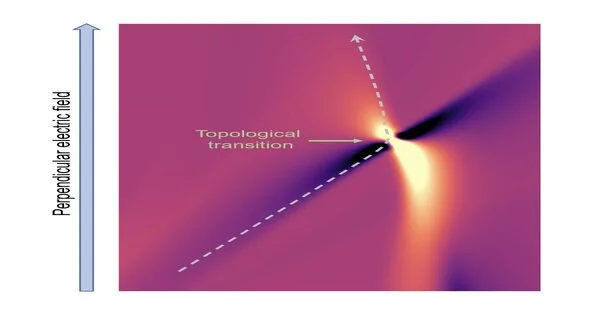
The Berry arch dipole (BCD) of TDBG, showed through variety. The dim purple tone shows a negative BCD, while the dazzling yellow tone demonstrates a positive BCD. By expanding the greatness of the opposite electric field, we can cross along the ran bolt to recognize the sign difference in the BCD. Sign difference in BCD occurs because of a topological change.
“Generally, the Hall voltage creates opposite to the progression of current when an outside attractive field is applied opposite to the example’s plane.” Sinha made sense of. “Curiously, the spearheading hypothetical work of Sodemann and Fu demonstrated the way that one can likewise have a Hall voltage without an attractive field even in non-attractive materials because of topological groups, and we estimated this voltage.”
Consolidated impact of a nonzero Berry curve and limited quantities of strain in contorted bilayer graphene framework can lead to what is known as the “Berry ebb and flow dipole.” This extraordinary estimation creates a nonlinear Hall voltage that scales quadratically with the current applied to a material example.
“We applied a low-recurrence current and estimated the Hall voltage at two times the recurrence of the applied current to distinguish the nonlinear Hall voltage,” Sinha said. “Then, we utilized a scaling examination to distinguish a sign change in the Berry shape dipole, demonstrating a topological stage progress.”
Topological stage advances are staggeringly challenging to tentatively distinguish. In any case, numerous hypothetical and trial studies have as of late alluded to a change in the geography of the groups of curved twofold bilayer graphene. The new work by the group expresses an immediate impression of this stage change in a trial setting.
“Utilizing transport estimations, we identified this topological progress straightforwardly through a sign change in the Berry shape dipole,” Sinha made sense of. “This gives us an exploratory handle for testing the band’s mathematical material science and topological stage advances at the same time.”
The discoveries accumulated by this group of analysts could have vital ramifications for the investigation of topological stage changes in contorted two-fold bilayer graphene. Later on, the techniques they utilized can help in distinguishing topological advances in different materials and frameworks.
“A short-term heading for our work can be involving our procedure to delineate the stage change as a component of a contort point or stacking request,” Sinha added.Likewise, we trust our technique will likewise be copied in other 2D or even 3D materials to portray comparable topological stage advances. By and large, the exploration interest in nonlinear Hall impacts is increasing because of its many benefits, one of which is examining the band mathematical and topological properties of materials. We should keep a watchful eye on the intriguing roads that nonlinear impacts can access, as they unfurl.
More information: Subhajit Sinha et al, Berry curvature dipole senses topological transition in a moiré superlattice, Nature Physics (2022). DOI: 10.1038/s41567-022-01606-y