At the core of each and every resonator—be it a cello, a gravitational wave finder, or the radio wire in your PDA—there is a lovely piece of math that has been unacknowledged until now.
Yale physicists Jack Harris and Nicholas Read have known this since they began looking for gaps in their data.
In another concentrate in the diary of Nature, Harris, Read, and their co-writers depict a formerly obscure trait of resonators. A resonator is any item that vibrates at a particular arrangement of frequencies. They are pervasive in sensors, devices, instruments, and different gadgets, where they are utilized to create, enhance, or identify vibrations at explicit frequencies.
The new trademark, the Yale group tracked down results from conditions that any secondary school polynomial math understudy would perceive, yet which physicists had not appreciated as an essential rule of resonators.
“The resonances round one another. It’s fantastic. It means that every time you tune an instrument, you’re braiding. And if you tune it so that two of the resonances remain equal, you’re creating a knot.”
Yale physicists Jack Harris
It is this: If you make a chart of how the resonator’s frequencies change while you “tune” the resonator—by shifting its properties in practically any manner—the diagram will show meshes and bunches.
“The resonances wind around one another.” It’s great, “Harris said.” “It implies that any time you tune an instrument, you’re making a mesh.” What’s more, assuming you tune it so you keep two of the resonances equivalent, you’re making a bunch. “
Harris is a test physicist. His meat and potatoes is investigating the manner in which geography and quantum mechanics impact sound and light. Frequently, he leads tests involving resonators that trap light or sound in actual pits.
However, in spite of the cutting-edge nature of the work, there are analogs to working with a lot easier instruments.
“To know every one of the manners in which it can vibrate, you’re doing likewise in my lab,” Harris said. “It’s the physical science of vibration.”
A couple of years prior, Harris was attempting to see a few inquisitive elements that showed up in his information when he was tuning a pit. He went to his partner, Read, the Henry Ford II Professor of Physics and teacher of applied material science and math at Yale.
Peruse made sense that these elements were meshes and were just articulations of a key numerical rule. “Yet, when he made sense that our information ought to contain trefoil hitches, I was snared,” Harris said.
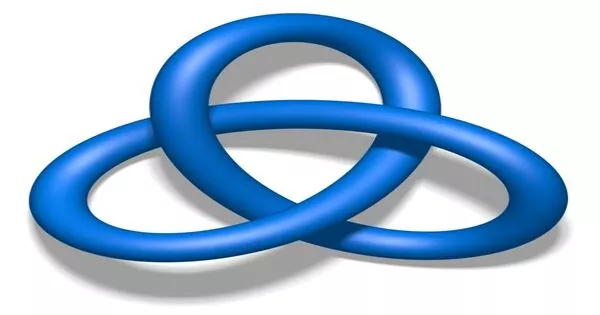
A trefoil tie is a figure tracked down in the iconography of many societies. It is likewise tracked down in the fine art of M.C. Escher. Bunches of this kind are natural to mathematicians, yet they don’t frequently manifest in material science.
Harris and Read planned a trial in which they tuned three frequencies of a resonator and did, for sure, notice the anticipated twists and bunches.
The revelation, while essential for math, may be helpful for physicists and designers. “It’s a possibly useful asset to realize that frequencies can mesh in a resonator,” Harris said. “That is on the grounds that a mesh is a topological item, meaning it doesn’t change its fundamental person assuming you twist it a piece.” Except when you truly mess it up, it remains a mesh. This is a unique sort of power that we think can be utilized to forestall mistakes in applications that depend on precisely tuning resonators. “
More information: Yogesh S. S. Patil et al, Measuring the knot of non-Hermitian degeneracies and non-commuting braids, Nature (2022). DOI: 10.1038/s41586-022-04796-w